Table of Contents
Thermal radiation
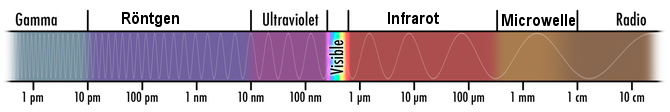
 Every object gives off thermal radiation - this can easily be seen in a thermographic image (the face and hand, which are both warm, emit the most heat). Thermal radiation is the transfer of energy by electromagnetic waves: as we have already shown, above all, thermal energy is the random movement of molecules; these are electrically charged. Moving electric charges are a source of electromagnetic waves; their spectrum ranges from extremely low frequencies (long waves) to radio and broadcasting frequencies and mobile radio bands as well as microwaves, infrared, visible light up to ultraviolet, x-ray and the “hard” γ-rays (see illustration; nm = nanometre = 10-9 m = 0.000001 mm; dimension is on the scale of an atomic diameter).
Every object gives off thermal radiation - this can easily be seen in a thermographic image (the face and hand, which are both warm, emit the most heat). Thermal radiation is the transfer of energy by electromagnetic waves: as we have already shown, above all, thermal energy is the random movement of molecules; these are electrically charged. Moving electric charges are a source of electromagnetic waves; their spectrum ranges from extremely low frequencies (long waves) to radio and broadcasting frequencies and mobile radio bands as well as microwaves, infrared, visible light up to ultraviolet, x-ray and the “hard” γ-rays (see illustration; nm = nanometre = 10-9 m = 0.000001 mm; dimension is on the scale of an atomic diameter).
Illustration: the electromagnetic spectrum with the visible range highlighted. The wavelength has been plotted on a logical scale on the x-axis (source: NASA).
| Electromagnetic wave spectrum | ||||
|---|---|---|---|---|
| Wavelength | Typically | Description | Frequency | Application |
| $\lambda$ / m | $f$ / Hz | |||
| $10^4 - 10^0$ | 3 m | Radio waves (LW - UKW) | $3 \cdot 10^4 - 3 \cdot 10^8$ | Broadcasting |
| $10^0 - 10^{-4}$ | 12 cm | Microwaves | $3 \cdot 10^8 - 3 \cdot 10^{12}$ | Mobile telephones LTE-2.6 GHz band |
| $10^{-4} - 10^{-6}$ | 8,7 µm | Infrared (IR) (“thermal radiation”) | $3 \cdot 10^{12} - 3 \cdot 10^{14}$ | Radiation from heaters |
| $4-7 \cdot 10^{-7}$ | 520 nm | Visible light 🌈 | approximately $4-8 \cdot 10^{14}$ | Human eye |
| $10^{-7} - 10^{-9}$ | 13,5 nm | Ultraviolet (UV) | $3 \cdot 10^{15} - 3 \cdot 10^{17}$ | UV light e.g. lithography |
| $10^{-9} - 10^{-11}$ | 0.1 nm | X-rays | $3 \cdot 10^{17} - 3 \cdot 10^{20}$ | Medical “screening”, CT scans |
| $10^{-11} - ... (0)$ | 1 pm | $\gamma$-rays | $3 \cdot 10^{20} - $ very high | Mössbauer spectroscopy |
Physically, these waves differ only due to their wavelengths (or frequency). However, there are large differences e.g. with regard to the “transmitting” technology, biological effect 2), sensation and technical application.
Radiation measurement
…which describes the behaviour of the radiating body: radiant power $˙Q$
of a body: total energy radiated per second with the unit watt W. Specific radiation $˙q$: radiant power per unit of area of the radiating surface. The contribution of an area element dA to the total radiation is given by d$˙Q = ˙q dA$, the measurement unit is $[˙q]$ = W/m². The radiation energy can be distributed over a whole range of wavelengths. The radiation concentrated on a wavelength is described by the spectral specific radiation $˙qf$ . This value indicates the amount of energy per unit area that is radiated in the wavelength interval between $f$ and $f + df$. The specific radiation results as the sum (integral) of the spectral specific radiation over all wavelengths.
The behaviour of a surface relating to radiation is described by the properties absorptivity, reflectivity, transmissivity and emissivity. If the radiation energy per unit of time and area is indicated as $˙q$ then the following properties can be defined:
Reflectivity:
${\displaystyle \rho = \frac {\dot{q}_r}{\dot{q}_i} } $
where r stands for reflected radiation and i for incident radiation.
Transmissivity
${\displaystyle \tau = \frac {\dot{q}_t}{\dot{q}_i}} $
(“passed through” = “transmitted” radiant power (t) divided by incident radiant power)
Absorptivity
${\displaystyle \alpha = \frac {\dot{q}_a}{\dot{q}_i}} $
(absorbed radiant power (a) based on incident radiant power)
The law of energy conservation also applies here
$\rho + \tau + \alpha =1 . $
For real bodies, $ρ$,$τ$ and $α$ are often highly dependent on the wavelength. For example, a glass pane has a high transmissivity for visible light (“short wavelengths”), and on the other hand a high absorptivity 3) for infrared radiation (which in building physics is called “longwave radiation”).
The so-called “black body” 4) was used as an ideal body for formulating radiation laws. It is defined by the fact that its absorptivity for all wavelengths is equal to 1, i.e. it absorbs all incident radiation completely. Nothing is reflected or transmitted. It turns out (see further on: radiation experiments) that the radiation properties of a body are closely linked to the absorption characteristics. An ideal absorber 5) is thus also an ideal emitter.
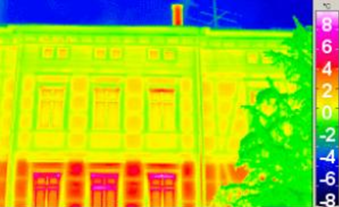
Illustration: with sensors that are sensitive in the infrared spectral range, it is possible to depict thermal radiation coming from objects in our surroundings (thermographic camera). Even a very cold object still emits thermal radiation: in the thermographic image the tree in the foreground is still recognisable at a temperature of -1 to -2 °C. The sky also radiates heat at -6°C (this is called “atmospheric counter-radiation”; this actually comes from the Earth's atmosphere, mostly from the H2O and CO2 molecules). The chimney at the top radiates more heat energy. Thermographic imaging is also used in space research: here is an interesting link about infrared astronomy: Infrared: More Than Your Eyes Can See. By the way: even the extremely bitterly cold outer space (-270°C) radiates microwaves, this radiation forms what is known as the Cosmic Microwave Background.
Against the backdrop of the images taken with the thermographic camera (thermography), here are three observations of paramount importance - we are often unaware of this because these images are quite similar to the images of visual photographs, especially if these are black and white pictures:
- What we see with the thermographic camera (sensitive in the range of ca. 5 to 17 µm) is thermal radiation which is produced by the observed objects - these are 6) inherent radiation emitters/self-radiators, like e.g. the visible solar disc, an incandescent bulb or a glowing radiant heater; the differences between the mentioned emitters/radiators lie solely in their temperature and therefore in the frequency range in which the major part of the electromagnetic waves is generated. In contrast, in “normal light” we see mostly radiation that is reflected by the surfaces on which the light falls (except in the case of particularly outstanding light “sources”). Thermal radiation therefore cannot simply be “switched off”; with an infrared/night vision camera a hunter can see game at night due to its own inherent radiation!
- The specific radiation of the objects around also us is by no means “small” in comparison with other energy flows 7) . Here we note that at normal temperatures of our surroundings, area-specific radiation is between 350 and 550 W/m². Thermal radiation abounds in the space around us; we can feel this radiation with the heat sensors in our skin - but we are not even aware that this is thermal radiation which we can sense. Against this background, some of us might certainly remember a late evening walk in summer, in the course of which we passed by a dark coloured solid brick wall, and we could clearly perceive very warm radiation coming from it even though a cool breeze prevailed. We can also feel this radiation coming from the glowing embers of a camp fire 8).
- And of course, thermal radiation is also reflected from the surfaces surrounding our bodies: this is usually diffuse because the surfaces are rough; this is again quite similar to light in the visual spectral range; glass panes are smooth, as if polished, and that is why the reflection of other bodies that are radiating heat can be seen in these. However, there is still a difference to the environment in visible light: for the mid infrared and far infrared range the reflectivities of the surfaces are usually very low 9) , in a range between 2% and 15%, the only exceptions being metallic surfaces which act as mirrors as usual also for thermal radiation. So while at first glance everything in the infrared range (largely) appears to look something like that in the visual/visible range, on closer examination there are significant differences.
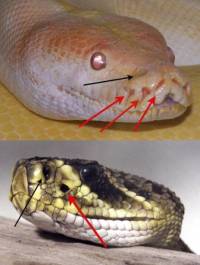
Small infrared cameras, particularly as USB devices, are available at affordable prices today. These usually still have a low spatial resolution today, but even so, this allows our surroundings to be experienced in a completely different spectral range. Many things can be directly visualised with these cameras. Besides having a whole range of useful technical applications for diverse purposes, this also has an immense educational value, since it shows us that besides the world we know about through the senses given to us, there are also some totally different channels of perception with which further peculiarities of our world are discernible which would otherwise elude us. What is interesting is the fact that the heat sensors of some animals have developed further into more sensitive sensory organs (pit organs of snakes, see illustration on the right: this serves a purpose for these nocturnal hunters). It is also interesting that so far we know of no other species which uses this sense with a higher resolution similar to the eye).
In Passipedia there are a number of documents in which infrared images of buildings and systems are shown (from the inside and the outside). A lot can be learned about the mechanisms of heat transport and the more or less effective solutions of the construction industry from these (as an example, here is the link to a thermographic inspection of an interior insulation measure: Interior insulation is better than you think (German only).
Table with typical absorptivities (=emissivities)
Except for ice and water, the surfaces listed here (for layers > 0.5 mm) are opaque 10) for heat radiation 11) ; this also applies for the material “glass” (normal float glass) which is predominantly absorptive in the infrared range and only 10 % is reflective. For this reason, heat radiation cannot directly enter through normal window glass; instead, heat flow “passes through” in this way: heat radiation coming from the room is absorbed at the glass surface on the room side, heat is thus supplied to the pane. Due to heat conduction, this heat is transferred further to the outer surface of the pane and then emitted again here (or transferred to the fluid on the other side also due to heat conduction).
| Material (surface temperature in °C) | Emissivity 12) =Absorptivity in the spectral range 5 to 18 µm |
|---|---|
| Aluminium, non-oxidised | 0.03 |
| Aluminium, heavily oxidised | 0.20 |
| Aluminium, highly polished | 0.09 |
| Cotton | 0.77 |
| Concrete | 0.93 |
| Ice or water with smooth surface (0°C) | 0.97 |
| Chromium | 0.08 |
| Iron, bright polished | 0.20 |
| Iron, heavily oxidised | 0.88 |
| Stainless steel, polished sheet metal | 0.18 |
| Enamel | 0.90 |
| Paint (e.g. emulsion paint on wallpaper) | 0.88 to 0.96 |
| Glass | 0.90 |
| Gypsum plaster (20°C) | 0.94 |
| Gold, mirror-polished | 0.02 |
| Rubber | 0.89 to 0.94 |
| Wood | 0.82 to 0.92 |
| Candle soot | 0.95 |
| Heat sink/cooling element, black, anodised | 0.98 |
| Scynthetics: PVC, polystyrene, glass fibre laminate … | 0.94 |
| Copper, polished | 0.03 |
| cCopper, heavily oxidised | 0.77 |
| Pcaint, (radiator), black, matt | 0.94 to 0.98 |
| Paper, matt, different colours | 0.92 to 0.94 |
| Paper, white, different types of gloss | 0.76 to 0.93 |
| Masonry; brick; roof tile; standard exterior plaster | 0.91 to 0.94 |
| Oil paints (different colours) | 0.92 to 0.96 |
| Porcelain | 0.92 |
| Silver, polished | 0.03 |
| Steel, cold-rolled | 0.7 to 0.85 |
| Wallpaper (“normal”, e.g. wood chip wallpaper) | 0.85 to 0.93 |
| Brick, mortar, plaster | 0.93 |
| Zinc, oxidised | 0.11 to 0.60 |
| Zinc, polished | 0.04 to 0.05 |
Continue to radiation laws 🌡️
back to section on heat transport (German only) 🌡️
Back to Building Physics Basic Course - Heat - Overview 🌡️